How reduction formulas work in trigonometry. Reduction formulas, mnemonic rule, proof, examples. Problems with reduction formulas for independent solution

And another problem B11 on the same topic - from the real Unified State Examination in mathematics.

Task. Find the meaning of the expression:

In this short video tutorial we will learn how to apply reduction formulas for solving real problems B11 from the Unified State Examination in mathematics. As you can see, we have two trigonometric expressions, each containing sines and cosines, as well as some pretty brutal numerical arguments.

Before solving these problems, let's remember what reduction formulas are. So, if we have expressions like:

Then we can get rid of the first term (of the form k · π/2) according to special rules. Let's draw a trigonometric circle and mark the main points on it: 0, π/2; π; 3π/2 and 2π. Then we look at the first term under the sign of the trigonometric function. We have:

1. If the term we are interested in lies on the vertical axis of the trigonometric circle (for example: 3π/2; π/2, etc.), then the original function is replaced by a co-function: sine is replaced by cosine, and cosine, on the contrary, by sine.
2. If our term lies on the horizontal axis, then the original function does not change. We simply remove the first term in the expression and that’s it.

Thus, we obtain a trigonometric function that does not contain terms of the form k · π/2. However, the work with reduction formulas does not end there. The fact is that our new function, obtained after “discarding” the first term, may have a plus or minus sign in front of it. How to identify this sign? Now we'll find out.

Let's imagine that the angle α remaining inside the trigonometric function after transformations has a very small degree measure. But what does “small measure” mean? Let's say α ∈ (0; 30°) - this is quite enough. Let's take an example of the function:

Then, following our assumptions that α ∈ (0; 30°), we conclude that the angle 3π/2 − α lies in the third coordinate quarter, i.e. 3π/2 − α ∈ (π; 3π/2). Let us remember the sign of the original function, i.e. y = sin x on this interval. Obviously, the sine in the third coordinate quarter is negative, since by definition, the sine is the ordinate of the end of the moving radius (in short, the sine is the y coordinate). Well, the y coordinate in the lower half-plane always takes negative values. This means that in the third quarter y is also negative.

Based on these reflections, we can write down the final expression:

Problem B11 - Option 1

These same techniques are quite suitable for solving problem B11 from the Unified State Examination in mathematics. The only difference is that in many real B11 problems, instead of a radian measure (i.e. numbers π, π/2, 2π, etc.) a degree measure is used (i.e. 90°, 180°, 270° and etc.). Let's look at the first task:

Let's look at the numerator first. cos 41° is a non-tabular value, so we can't do anything with it. Let's leave it like that for now.

Now let's look at the denominator:

sin 131° = sin (90° + 41°) = cos 41°

Obviously, this is a reduction formula, so the sine is replaced by a cosine. In addition, the angle 41° lies on the segment (0°; 90°), i.e. in the first coordinate quadrant - exactly as required to apply the reduction formulas. But then 90° + 41° is the second coordinate quarter. The original function y = sin x is positive there, so we put a plus sign in front of the cosine at the last step (in other words, we didn’t put anything).

It remains to deal with the last element:

cos 240° = cos (180° + 60°) = −cos 60° = −0.5

Here we see that 180° is the horizontal axis. Consequently, the function itself will not change: there was a cosine - and the cosine will also remain. But the question arises again: will plus or minus appear before the resulting expression cos 60°? Note that 180° is the third coordinate quarter. The cosine there is negative, therefore, the cosine will eventually have a minus sign in front of it. In total, we get the construction −cos 60° = −0.5 - this is a tabular value, so everything is easy to calculate.

Now we substitute the resulting numbers into the original formula and get:

As you can see, the number cos 41° in the numerator and denominator of the fraction is easily reduced, and the usual expression remains, which is equal to −10. In this case, the minus can either be taken out and placed in front of the fraction sign, or “kept” next to the second factor until the very last step of the calculations. In any case, the answer will be −10. That's it, problem B11 is solved!

Problem B14 - option 2

Let's move on to the second task. We have a fraction before us again:

Well, 27° lies in the first coordinate quarter, so we won’t change anything here. But sin 117° needs to be written (without any square for now):

sin 117° = sin (90° + 27°) = cos 27°

Obviously, before us again reduction formula: 90° is the vertical axis, therefore the sine will change to cosine. In addition, the angle α = 117° = 90° + 27° lies in the second coordinate quadrant. The original function y = sin x is positive there, therefore, after all the transformations, there is still a plus sign in front of the cosine. In other words, nothing is added there - we leave it like that: cos 27°.

We return to the original expression that needs to be calculated:

As we see, after the transformations, the main trigonometric identity arose in the denominator: sin 2 27° + cos 2 27° = 1. Total −4: 1 = −4 - so we found the answer to the second problem B11.

As you can see, with the help of reduction formulas such problems from the Unified State Examination in mathematics are solved literally in a couple of lines. No sine of the sum and cosine of the difference. All we need to remember is just the trigonometric circle.

There are two rules for using reduction formulas.

1. If the angle can be represented as (π/2 ±a) or (3*π/2 ±a), then function name changes sin to cos, cos to sin, tg to ctg, ctg to tg. If the angle can be represented in the form (π ±a) or (2*π ±a), then The function name remains unchanged.

Look at the picture below, it shows schematically when you should change the sign and when not.

2. The rule “as you were, so you remain.”

The sign of the reduced function remains the same. If the original function had a plus sign, then the reduced function also has a plus sign. If the original function had a minus sign, then the reduced function also has a minus sign.

The figure below shows the signs of the basic trigonometric functions depending on the quarter.

Calculate Sin(150˚)

Let's use the reduction formulas:

Sin(150˚) is in the second quarter; from the figure we see that the sign of sin in this quarter is equal to +. This means that the given function will also have a plus sign. We applied the second rule.

Now 150˚ = 90˚ +60˚. 90˚ is π/2. That is, we are dealing with the case π/2+60, therefore, according to the first rule, we change the function from sin to cos. As a result, we get Sin(150˚) = cos(60˚) = ½.

If desired, all reduction formulas can be summarized in one table. But it’s still easier to remember these two rules and use them.

Need help with your studies?

They belong to the trigonometry section of mathematics. Their essence is to reduce trigonometric functions of angles to a “simple” form. Much can be written about the importance of knowing them. There are already 32 of these formulas!

Don’t be alarmed, you don’t need to learn them, like many other formulas in a math course. There is no need to fill your head with unnecessary information, you need to remember the “keys” or laws, and remembering or deriving the required formula will not be a problem. By the way, when I write in articles “... you need to learn!!!” - this means that it really needs to be learned.

If you are not familiar with reduction formulas, then the simplicity of their derivation will pleasantly surprise you - there is a “law” with the help of which this can be easily done. And you can write any of the 32 formulas in 5 seconds.

I will list only some of the problems that will appear on the Unified State Exam in mathematics, where without knowledge of these formulas there is a high probability of failing in solving them. For example:

– problems for solving a right triangle, where we are talking about the external angle, and problems for internal angles, some of these formulas are also necessary.

– tasks on calculating the values ​​of trigonometric expressions; converting numerical trigonometric expressions; converting literal trigonometric expressions.

– problems on the tangent and the geometric meaning of the tangent, a reduction formula for the tangent is required, as well as other problems.

– stereometric problems, in the course of solving it is often necessary to determine the sine or cosine of an angle that lies in the range from 90 to 180 degrees.

And these are just those points that relate to the Unified State Exam. And in the algebra course itself there are many problems, the solution of which simply cannot be done without knowledge of reduction formulas.

So what does this lead to and how do the specified formulas make it easier for us to solve problems?

For example, you need to determine the sine, cosine, tangent, or cotangent of any angle from 0 to 450 degrees:

the alpha angle ranges from 0 to 90 degrees

* * *

So, it is necessary to understand the “law” that works here:

1. Determine the sign of the function in the corresponding quadrant.

Let me remind you:

2. Remember the following:

function changes to cofunction

function does not change to cofunction

What does the concept mean - a function changes to a cofunction?

Answer: sine changes to cosine or vice versa, tangent to cotangent or vice versa.

That's all!

Now, according to the presented law, we will write down several reduction formulas ourselves:

This angle lies in the third quarter, the cosine in the third quarter is negative. We don’t change the function to a cofunction, since we have 180 degrees, which means:

The angle lies in the first quarter, the sine in the first quarter is positive. We do not change the function to a cofunction, since we have 360 ​​degrees, which means:

Here is another additional confirmation that the sines of adjacent angles are equal:

The angle lies in the second quarter, the sine in the second quarter is positive. We do not change the function to a cofunction, since we have 180 degrees, which means:

Work through each formula mentally or in writing, and you will be convinced that there is nothing complicated.

***

In the article on the solution, the following fact was noted - the sine of one acute angle in a right triangle is equal to the cosine of another acute angle in it.

Lesson and presentation on the topic: "Application of reduction formulas in solving problems"

Additional materials
Dear users, do not forget to leave your comments, reviews, wishes. All materials have been checked by an anti-virus program.

Teaching aids and simulators in the Integral online store for grade 10
1C: School. Interactive construction tasks for grades 7-10
1C: School. We solve problems in geometry. Interactive tasks on building in space for grades 10–11

What we will study:
1. Let's repeat a little.
2. Rules for reduction formulas.
3. Conversion table for reduction formulas.
4. Examples.

Review of trigonometric functions

Guys, you’ve already come across ghost formulas, but you haven’t called them that yet. What do you think: where?

Look at our drawings. Correctly, when the definitions of trigonometric functions were introduced.

Rule for reduction formulas

Let's introduce the basic rule: If under the sign of the trigonometric function there is a number of the form π×n/2 + t, where n is any integer, then our trigonometric function can be reduced to a simpler form, which will contain only the argument t. Such formulas are called ghost formulas.

Let's remember some formulas:

• sin(t + 2π*k) = sin(t)
• cos(t + 2π*k) = cos(t)
• sin(t + π) = -sin(t)
• cos(t + π) = -cos(t)
• sin(t + π/2) = cos(t)
• cos(t + π/2) = -sin(t)
• tan(t + π*k) = tan(x)
• ctg(t + π*k) = ctg(x)

there are a lot of ghost formulas, let's make a rule by which we will determine our trigonometric functions when using ghost formulas:

• If the sign of a trigonometric function contains numbers of the form: π + t, π - t, 2π + t and 2π - t, then the function will not change, that is, for example, the sine will remain a sine, the cotangent will remain a cotangent.
• If the sign of the trigonometric function contains numbers of the form: π/2 + t, π/2 - t,
  3π/2 + t and 3π/2 - t, then the function will change to a related one, that is, the sine will become a cosine, the cotangent will become a tangent.
• Before the resulting function, you need to put the sign that the transformed function would have under the condition 0

These rules also apply when the function argument is given in degrees!

We can also create a table of transformations of trigonometric functions:

Examples of using reduction formulas

1. Transform cos(π + t). The name of the function remains, i.e. we get cos(t). Let us further assume that π/2

2. Transform sin(π/2 + t). The name of the function changes, i.e. we get cos(t). Next, assume that 0 sin(t + π/2) = cos(t)

3. Transform tg(π + t). The name of the function remains, i.e. we get tan(t). Let us further assume that 0

4. Transform ctg(270 0 + t). The name of the function changes, that is, we get tg(t). Let us further assume that 0

Problems with reduction formulas for independent solution

Guys, convert it yourself using our rules:

1) tg(π + t),
2) tg(2π - t),
3) cot(π - t),
4) tg(π/2 - t),
5) cotg(3π + t),
6) sin(2π + t),
7) sin(π/2 + 5t),
8) sin(π/2 - t),
9) sin(2π - t),
10) cos(2π - t),
11) cos(3π/2 + 8t),
12) cos(3π/2 - t),
13) cos(π - t).

How not to memorize reduction formulas.

When solving trigonometric equations or performing trigonometric transformations, the first step is to minimize the number of different arguments of trigonometric functions. To do this, you need to bring all the angles to the angles of the first quarter, using reduction formulas. I want to introduce you to a mnemonic rule that allows you to avoid memorizing. This rule is jokingly called the "Horse Rule".

In this VIDEO TUTORIAL I will tell you how to use this rule: reduce the trigonometric function of an arbitrary angle to the angle of the first quarter, freeing yourself from the need to remember reduction formulas:

So, " horse rule " sounds like this:

If we plot the angle from vertical axis, the horse says “yes” (we nod our head along the OY axis) and the reducible function changes its name: sine to cosine, cosine to sine, tangent to cotangent, cotangent to tangent.

If we plot the angle from horizontal axis, the horse says “no” (we nod our head along the OX axis) and the reduced function does not change its name.

The sign on the right side of the equality coincides with the sign of the reducible function on the left side of the equality.

Here are some examples of using reduction formulas:

1 . Find the meaning of the expression:

1. Select the whole part in the fraction:

2. Since the period of the function is equal to , let’s highlight the “idle speed”:

Now our argument is in the range from zero to , and it's time to apply the "horse rule":

To get to the point corresponding to the rotation angle by , we first make a rotation by radians, and then from this point we plot an angle of radians:

We plotted the angle from the horizontal axis (the horse says “no”) - it does not change its name, the angle is located in the third quarter, in which the cosine is negative, therefore the reduced function is negative. We get:

2 . Find the meaning of the expression:

Let's look at each function separately:

We first rotate by a radian and then make an angle of 1 radian from the vertical axis in the negative direction and end up in the third quarter:

Consequently, the reducible function changes its name, the reducible function is greater than zero (the tangent of the third quarter angle is greater than zero): .

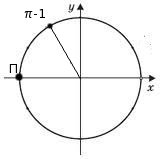First we make a turn by a radian, and then from this point we move by 1 radian in a negative direction. We set aside an angle of 1 radian from the horizontal axis (the sine does not change its name) and find ourselves in the second quarter, in which the sine is greater than zero: